Introduction to Music Theory

The word ‘theory’ comes from the ancient Greek word for contemplation. As a young musician, I had always imagined this 6-letter word to contain information and concepts that I could not begin to understand. Why bother when all I wanted to do was play boogie-woogie or rock-and-roll. From the start I believed music was a free form of expression that is unique based on an experience. Even classical musicians must interpret and experience the feeling of a work they can only read. The truth is that music is so universal and so experiential that it seems ridiculous to attempt to create a single set of rules to confine, and possibly corrupt, something so pure.

However, as I matured, I realized I needed a written system to note down my musical ideas. Of course I could easily record on my computer, but my computer would soon become a graveyard of incomplete or barely finished thoughts. I also once tried to develop my own musical notation, which lasted perhaps six months before I put down that effort. As a guitarist, I can’t say I even liked tablature that much. Thus, with a groan, I committed myself to comply with standard musical notation. I had always been able to read to some degree, but my writing never fit accurately. I spent hours a day for a year or two sightreading music and then one day something clicked, as though a cloud had lifted from my eyes, and I began to see how truly clever music is if viewed with a little bit of simple mathematics. From that day forward, I had been able to enjoy very much the ‘contemplation’ aspect of music theory and the small secret that helped me I would like to share with you so you may enjoy ‘contemplating’ music as much as playing and listening.

Proportions: E Pluribus Unum

Something they teach in grade school is how to make change. If I wish to buy an apple for fifty cents ($0.50) and I only have a $1 dollar bill, the cashier may be inclined to give me two quarters change. This is because, for US currency, one can traditionally achieve a more exact value for goods and services where $1 is equal to 2 half-dollars is equal to 4 quarters, and so on with dimes (10) and nickels (20), resulting in pennies (100) as the lowest form of value. It can also be said that a half-dollar, a coin worth 50 cents, is proportionate to the dollar by ½; similarly, a quarter is proportionate to the dollar by ¼. And so it is that governments and businesspeople solved a problem of better accounting by issuing currency proportional to one dollar. This system may be becoming outdated due to inflation, but the general concept of breaking ONE into multiple parts is key to the following foundations of music theory.

Again, please humor the simplicity of this – it is my wish to maintain simplicity and ease of understanding, however, thinking in proportions helps well beyond money, well beyond music, and I strongly encourage young readers to embrace conveying numbers in terms of ratios and proportions.

The Proportion of Pitch: The First Concept

The jury may still be out on who actually developed the theory on pitch, but I read it as Pythagoras and I always like imagining this bearded old dude doing some tinkering that would ultimately lay the foundations of western music for the rest of time.

The story goes something like this: Pythagoras was chilling in his yard one afternoon when one of his friends showed up with a gift. He was a musician and was making room for new gear so he thought his buddy Pythagoras would enjoy having a nice sized bell. Pythagoras was overjoyed (always secretly wanting to be a musician) and thanked his friend very much. While he enjoyed listening to the deep resonant sound that emanated from within the object as he struck, he couldn’t help but wonder why it made that particular sound. So, being a math guy, he started taking measurements and running tests. He went back to his workshop and made a bell exactly half (½) the size of the original. When he struck both at the same time, both bells produced the same note just the small one was higher than the larger one. That day he discovered what we call an octave and sure enough if he made a bell twice the size of the original, he would have heard the same note but a level lower (Figure 1).

Figure 1: Pythagoras’s Bells – to illustrate how size, or diameter (red line), relates to pitch, the original bell is on the left while a bell half that size (its octave) is on the right. A bell 2/3 of the original bell (the perfect fifth) is in the middle.

(Bells)

He pondered this, ‘I can make many bells by halving or doubling its size, but they all sound the same! How can I make bells that sound different, yet harmonious?’ With this he tried the next sensible thing, experimenting with the size of bells proportionate to the original. He produced many bells that, when played with the original, just did not sound right; something we today might call dissonant harmonies. However, when he played a bell with the proportionate size of the bells rang together in such a pleasing way; today what we call a consonant harmony. Immediately, inspiration struck, and he began to make a series of bells by multiplying together with either ½ or 2 (accounting for octaves) and developed the scale of 12-tones that we today call the chromatic scale (Figure 2).

Figure 2: Chromatic Scale – 12-tones of western music scales – starting on C (key of C) -

Of course, one might read this as a scale based on perfect fifths, which is more formally the ratio , however the math is not as important as understanding that all the notes you will play on any (western) instrument are harmonically related because they are proportional to one another! In fact, a scale would be that series of notes harmonically related to the first note; as in Pythagoras’s case, the original bell size would have served as his root note. Another word for this is diatonic, or ‘through tone’, and a purely diatonic scale, where all intervals, or notes, are consonant with the root note consists of seven notes. One can achieve this mathematically (using ratios) or by referring to the chromatic scale as a series of semi-tones and whole-tones. Conveniently, two semi-tones (half-steps) equal one whole-tone (whole-step). Also conveniently, since the tones repeat themselves at all octaves, only seven letters (A-G) needed to be applied to indicate important intervals of the diatonic scale (Figure 3).

Figure 3: Major diatonic scale - Key of C – notes: C-D-E-F-G-A-B-C (no sharps or flats) – the chromatic scale above can be referred to visualize the steps used to achieve this scale – remember 1 whole=2 half steps.

Not to be a broken record, but scales are wonderful BECAUSE they are made of harmonically related tones. Just playing the individual notes you can easily work out a melody, improvise over chords, or build more interesting chords and harmonies using this framework. It is the magic of this relationship that inspired the great jazz pianist Thelonious Monk to say, “there are no wrong notes”. Again, all this, how scales are used, will be discussed in later lessons, but it is of the utmost importance now to embrace the concept of how scales are built, which is nothing more than a small pattern using proportions.

If you are wondering about the size of the original bell that all these other notes are related to, it is useful to understand we most often tune our instruments to an A of 440 hertz (Hz), or cycles per second. One can imagine the bell’s vibrations as a wave cycling back-and-forth 440 times a second, but this, as well, is too beyond the scope of this lesson.

The Proportion of Time: The Second Concept

Boy howdy, that sure did seem to get thick at times! This section promises to bring home a much simpler concept, yet just as important, and is also entirely indebted to the wonders of proportions!

Figure 4: Analogy of how a Dollar is divided into Cents a Measure of music is divided into equal parts filled in with either notes or rests.

The question we will venture to answer is: what notation is used to convey rhythm? We saw briefly that notes, or pitch, were written as dots on a staff of 5 lines, and if the dot was outside of the staff a ledger line would be used to indicate the location of the pitch. Thus, the lines served to separate the tones, so we know which note to play, but how do we know how long we play the note?

Well, if we go back to our example of money, perhaps we can better illustrate how different shapes of notes (and rests) are added together to equal one (1) measure, or bar. Remember, 2 half-dollars, as well as 4 quarters, equal 1 dollar, therefore, similarly, if we think of a measure as a dollar, we can come up with different notes that add up to one measure (Figure 4).

Easy right? A careful eye might notice the first staff in Figure 4 has a filled in rectangle and not a circle as in the two measures below it. That black rectangle is known as a rest, which is a symbol that can have the same value as any note, but is not played, just counted (Figure 5).

	Figure 5: Notation for Note and Rest values relative to one another.

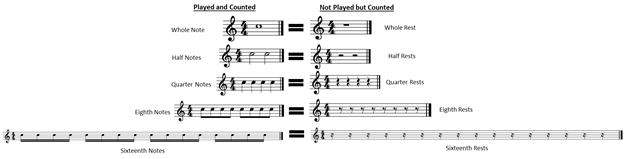
Again, a careful eye will be able to follow how each note or rest divides by 2 as one reads downwards in Figure 5. One may also notice after quarter notes/rests, the next smaller value is eighth notes/rests, and even furthermore, the smaller value after that are sixteenth notes/rests. Where a dollar stops dividing by 2 after quarters, musical notation stops dividing by 2 at the sixty-fourth note! However, a note that small is rarely used, only on special occasions when you need to play something really fast. Thus, Figure 5 only intends to demonstrate the continuous breakdown of a whole value to a sixteenth value to give you an idea of how a measure is divided into proportionate values.

One of reasons why sixty-fourth notes are rarely used has a lot to do with the two numbers you see stacked together on the left side of the measure. Of course, the little curly line is called a clef, a treble clef to be exact, but there are many other designs for a clef and each indicate a starting pitch to inform the musician whether the notes are low notes or high notes. Where the clef is important to the pitch, the stacked numbers, or time-signature, is important to how the musician counts the notes and rests. All the figures above have 4/4 (said, “four-four”) time, which communicates that each measure has 4 beats, as indicated by the number 4 on top, and a quarter note is equal to a beat, as indicated by the number 4 on the bottom. While the time signature can also be changed to include a different number of beats per measure or which note “gets the beat”, the 4/4-time signature is so common its actually referred to as ‘common-time’. Again, the topics of clefs and time signatures will be covered in more depth in later lessons, but, for now, I believe we have demonstrated how proportions, and dividing one thing into smaller parts, is central to understanding music!

Closeout

Well guys, we’ve covered quite a bit of music theory, and the good news is that the rest of it is all details. In the lessons to follow we will further discuss the pitch side of things: clefs, scales, modes, arpeggios, chords, and inversions; as well as the rhythm side of things: tempo, time-signatures, and counting. As we do, we will introduce more music theory terms that help us navigate a piece. We will further venture into guitar technique as pitch relates to fingering positions and rhythm relates to strumming and we will discuss how tablature notation is read and used to help guitarists move more intuitively through a piece of music. Rome wasn’t built in a day, and neither should you feel pressured to understand everything all at once. Take your time, ask questions, use these resources and any other to fill in the blanks and you’ll be all set in no time!